管理人オススメコンテンツはこちら
「投資界のラーの鏡=IRR|表面利回りに騙されるな!退職金運用の落とし穴」
〜前回のつづき〜
●退職金を個人年金保険で運用すると結局いくらになるのか?
もう一度
この年金保険の設計を
見てみましょう。
条件(1)60歳で1千万円全額を振り込む
条件(2)65歳から受給開始される(年額626,400円)
条件(3)受給期間15年間
条件(4)6年目以降年金は毎年5%ずつ増えていく
条件(5)年金の受取総額は1,140万円強
上記の前提条件で
この年金の最終利回りの
計算方法は分かりますか?
「だいたい利回りは
これぐらいかなぁ?」
と何となくの感覚で
考えてみて欲しいんですよ。
もしわからないという人は
計算方法を
知っておいて欲しいんです。
以前お話ししたことが有るんですけど
5年間の据え置き期間が有って
↓
その後給付の期間が始まって
↓
6年目以降から年金が増えていく
複雑といえば
複雑な部類の商品で
「複雑な金融商品はどうだったっけ?」
と言えば
何となく答えは
見えてる気がするんですけど
順を追って
最終利回りというのを
計算してみましょう。
過去にもお話ししたんですけど

(出典:https://www.akinonbiri.work/entry/dq6-play9)
真実を映し出す
『投資界のラーの鏡』と呼んでる
IRR関数というのを使って
利回りを計算してみましょう。
↓こちらの計算結果になりました。
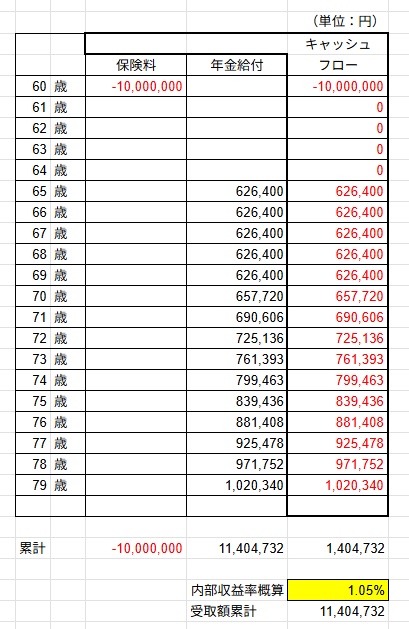
(IRR計算結果)
こんな感じになったんですけど
結論から言うと
複利計算で1.05%になりました。
条件(1)60歳で1千万円全額を振り込む
条件(2)65歳から受給開始される(年額626,400円)
条件(3)受給期間15年間
条件(4)6年目以降年金は毎年5%ずつ増えていく
条件(5)年金の受取総額は1,140万円強
という事でこんな結果になりました。
「細かい計算がよくわからない!」
「萎えるー!」
「数字嫌ーい!」
という人は1千万円かけたものが
20年間かけて1040万円になった
という事だけ
覚えておいてもらったら
とりあえずOKです。
という事で
最終利回りが約1.05%。
IRRを使った
複利の算定形式は非常に便利で
この計算がパッと出来ると
ぼったくり保険に騙される事が
無くなります。
大体表面利回りとか
保険会社にとって
都合のいい計算方法で
計算されてる数字を
見せられる事が多いので
このIRRを使って
本当の利回りを計算出来ると
こういうぼったくり保険に
騙されるという事が
なくなってくると思うので
知らなかったという人は
是非マスターして下さい。
・個人年金保険
・貯蓄型終身保険
・学資保険
返戻率〇%という
非常に魅力的に見える数字に
惑わされる事なく
本当の複利を自分で
一回でいいから計算してみる
というのが非常に大事です。
〜〜〜つづく〜〜〜
Special Thanks college president Ryo.
●おまけ
≪≪Chat-GPTくんによる要約→perplexityちゃんによる文章まとめ≫≫
退職金1,000万円を個人年金保険で運用した場合、5年間の据え置き後、15年間にわたり年金を受け取るプランを例に、実際の利回りをIRR(内部収益率)で計算すると年1.05%となります。
表面上の返戻率は約114%と魅力的に見えますが、実際の利回りは低く、インフレ率を考慮すると資産価値が目減りする可能性があります。
金融商品は、単純な返戻率や保険会社の提示する数字だけでなく、IRRを使って時間価値を考慮した実質利回りを自分で計算することが重要です。これにより、見かけだけの高利回りに惑わされず、より合理的な資産運用判断ができるようになります。
Citations:
[1] https://www.hokende.com/life-insurance/pension/basic_info/select_return_rate
[2] https://money-career.com/article/636
[3] https://www.f-l-p.co.jp/knowledge/62957
[4] https://www.jili.or.jp/knows_learns/q_a/tax/568.html
[5] https://adviser-navi.co.jp/insurance/column/2238/
[6] https://life.insweb.co.jp/nenkin/yotei-riritsu.html
[7] https://hoken-room.jp/pension/11666
[8] https://www.daiwa.jp/lp_dc/ideco/column/article_130/
[9] https://www.dai-ichi-life.co.jp/promotion/stepjump/01/index.html
[10] https://xtech.nikkei.com/it/pc/article/knowhow/20130627/1096128/
≪≪Chat-GPTくんによる英訳≫≫
~Continuation from Last Time~
【How much will your retirement bonus eventually amount to if you invest it in a personal pension insurance plan?】
Let’s take another look
at the design of this pension insurance.
Conditions:
(1) Pay in 10 million yen in full at age 60
(2) Start receiving payments at age 65 (annual payout: 626,400 yen)
(3) Payout period: 15 years
(4) From the 6th year onward, the annual payout increases by 5% each year
(5) Total amount received: slightly over 11.4 million yen
Based on these assumptions,
do you know how to calculate the final return?
I’d like you to roughly estimate,
“Maybe the return is about this much?”
Even if you’re not sure,
I want you to at least understand how the calculation is done.
As I mentioned before,
– There is a 5-year deferral period,
– Then the payout period begins,
– And from the 6th year, the payouts increase each year.
It’s a fairly complex product.
When you hear “complex financial product,”
you might already have a sense of what the answer will be.
Let’s work step-by-step to calculate the final return.
As I’ve shared before,
there’s a tool called the IRR function —
what I call the “Mirror of Ra” of the investment world —
that reveals the truth.
Let’s use the IRR function to calculate the return.
↓ Here are the results from the IRR calculation.
In conclusion,
the compound annual return came out to about 1.05%.
(Conditions again:)
(1) Pay in 10 million yen in full at age 60
(2) Start receiving payments at age 65 (annual payout: 626,400 yen)
(3) Payout period: 15 years
(4) From the 6th year onward, payouts increase by 5% per year
(5) Total amount received: slightly over 11.4 million yen
Thus, we get this result.
If you think,
“I don’t understand the complicated math!”
“This is exhausting!”
“I hate numbers!”
— that’s totally fine.
Just remember:
10 million yen grew into about 10.4 million yen over 20 years.
That’s enough for now.
So again, the final return is about 1.05%.
Using the IRR method for calculating compound returns
is extremely useful.
Once you can quickly run this calculation,
you won’t fall for overpriced insurance products.
Insurance companies often present returns
calculated in ways that are favorable to them,
such as simple returns, not compound ones.
By using IRR to find the true return yourself,
you can avoid falling victim to overpriced products.
If you didn’t know this,
please make sure to master it.
Especially for:
– Personal pension insurance
– Whole life savings insurance
– Educational endowment insurance
Don’t be fooled by
seemingly attractive “return rates” advertised.
It’s crucial to calculate the real compound return yourself
— even just once.
Special Thanks OpenAI and Perplexity AI, Inc








